Sunday, December 22, 2024
Banking QuizQuant

Quantitative Aptitude: Mensuration Set 2

infoalfabanker
  1. The parameter of a square is equal to the perimeter of a rectangle of length 14 cm and breadth 20 cm. Find the circumference of a semicircle (approx.) whose diameter is equal to the side of the square.
    A) 32 cm
    B) 22 cm
    C) 30 cm
    D) 27 cm
    E) 19 cm
    View Answer
    Option D
    Solution:
    Parameter of square = 2 * (14+20) = 68cm
    So side of square = 68/4 = 17 cm
    So diameter of semicircle = 17 cm
    So circumference of a semicircle = πr = 22/7 * 17/2 = 27 cm
  2. There are two circles of different radius such that radius of the smaller circle is three – sevens that of the larger circle. A square whose area equals 3969 sq cm has its side as thrice the radius of the larger circle. What is the circumference of the smaller circle?
    A) 59 cm
    B) 56.5 cm
    C) 49.5 cm
    D) 65.5 cm
    E) 62 cm
    View Answer
    Option B
    Solution:
    Side of square = √3969 = 63 cm
    So radius of larger circle = 1/3 * 63 = 21 cm
    So radius of smaller circle = 3/7 * 21 = 9 cm
    So circumference of smaller circle = 2 * 22/7 * 9 = 56.5 cm
  3. A Birthday cap is in the form of a right circular cone which has base of radius as 9 cm and height equal to 12 cm. Find the approximate area of the sheet required to make 8 such caps.
    A) 3225 cm2
    B) 3278 cm2
    C) 3132 cm2
    D) 3392 cm2
    E) 3045 cm2
    View Answer
    Option D
    Solution:
    r = 9, h = 12
    So slant height, l = √(92+122) = 15 cm
    So curved surface area of a cap = πrl = 22/7 * 9 * 15 = 424 sq. cm
    So curved surface area of 8 such cap = 424*8 = 3392 sq. cm which is also equal to area of sheet required to make 8 such caps
  4. The barrel of a fountain pen is cylindrical in shape which radius of base as 0.7 cm and is 5 cm long. One such barrel in the pen can be used to write 300 words. A barrel full of ink which has a capacity of 14 cu cm can be used to write how many words approximately?
    A) 598
    B) 656
    C) 508
    D) 545
    E) 687
    View Answer
    Option D
    Solution:
    Volume of the barrel of pen = πr2h = 22/7 * 0.7*0.7 * 5 = 7.7 cu cm
    A barrel which has capacity 7.7 cu cm can write 300 words
    So which has capacity 14 cu cm can write = 300/7.7 * 14 = 545 words
  5. A vessel is in the form of a hemi-spherical bowl on which is mounted a hollow cylinder. The diameter of the sphere is 14 cm and the total height of vessel is 15 cm, find the capacity of the vessel.
    A) 1977.23 cm3
    B) 1999.45 cm3
    C) 1840.67 cm3
    D) 1950.67 cm3
    E) 1833.27 cm3
    View Answer
    Option D
    Solution:

    Diameter is 14, so radius is 7 cm
    Total height = 15 cm, so height of cylinder = 15-7 = 8 cm (because height of hemisphere is same as its radius)
    Capacity of vessel = volume of cylinder + vol of hemisphere
    So = πr2h + 2/3 *πr3
    = 22/7 * 7 * 7 * 8 + 2/3 * 22/7 * 7 * 7 * 7
    = 1232 + 718.67
    = 1950.67 cu cm
  6. A car has wheels of diameter 70 m. How many revolutions can the wheel complete in 20 minutes if the car is travelling at a speed of 110 m/s?
    A) 550
    B) 580
    C) 630
    D) 640
    E) 600
    View Answer
    Option E
    Solution:
    Radius of wheel = 70/2 = 35 cm
    Distance travelled in one revolution = 2πr = 2 * 22/7 * 35 = 220 cm
    Let the number of revolutions made by wheel is x
    So total distance travelled = distance travelled in one revolution * number of revolutions
    So total distance travelled = 220x cm
    20 mins = 20*60 seconds
    Speed of car = 220x/(20*60)
    So 110 = 220x/(20*60)
    Solve, x = 600
  7. A clock has its minute hand of length 7 cm. What area will it swept in covering 10 minutes?
    A) 32.17 cm2
    B) 35.67 cm2
    C) 45.45 cm2
    D) 41.23 cm2
    E) None of these
    View Answer
    Option B
    Solution:
    Length will be the radius, so r = 7cm
    Minute hand covers 360o in 60 minutes
    So in 10 minutes it covers = 60o
    Area of arc = angle it makes/360 * πr2
    So area covered = 60/360 * 22/7 * 7 * 7 = 25.67
  8. Find the area of shaded region (approximately) in the given figure if AB = 12 cm and BC = 9 cm with O being the centre of circle.

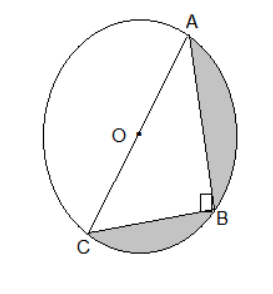
    A) 40 cm cm2
    B) 27 cm cm2
    C) 23 cm2
    D) 39 cm2
    E) 34 cm2

    View Answer
    Option E
    Solution:
    ABC forms a right angles triangle, so AC = √(9^2 + 12^2) = 15 cm
    So diameter of circle = 15 cm, so radius = 15/2 cm
    Area of semicircle = ½ * 22/7 * 15/2 * 15/2 = 88.39 sq cm
    Area of triangle = 1/2 * base * height = 1/2 * 9 * 12 = 54 sq cm
    So area of shaded region = 88.39 – 54 = 34
  9. The diameters of the internal and external surfaces of a hollow spherical shell are 10cm and 6 cm respectively. If it is melted and recasted into a solid cylinder of length 8/3 cm, find the diameter of the cylinder.
    A) 28√2 cm
    B) 14√2 cm
    C) 26√2 cm
    D) 18√2 cm
    E) 22√2 cm
    View Answer
    Option A
    Solution:
    External diameter of a sphere = 10 cm
    Internal diameter of the sphere = 6 cm
    Volume of the sphere = 4/3 π (R3 – r3)
    = (4/3) (22/7) (103 – 63)
    = (4/3) (22/7) (784)
    = 9856 / 3 cm3
    Height of the cylinder formed = 8/3 cm
    Let the radius of the cylinder be ‘r’ cm
    Volume of the cylinder = πr2h
    = 22/7 * r2 * 8/3
    = 22/7 * r2 * 8/3 = 9856 / 3
    r2 = 392
    r = 14√2 cm
    So Diameter of the cylinder = 2 x 14√2 =28√2 cm
  10. The radii of two cylinders are in the ratio 4 : 5 and their curved surface areas are in the ratio 3 : 5. What is the ratio of their volumes?
    A) 11 : 24
    B) 13 : 21
    C) 7 : 19
    D) 11 : 15
    E) 12 : 25
    View Answer
    Option E
    Solution:
    r1/r2 = 4/5
    CSA1/CSA2 = 2πr1h1/2πr2h2 = 3/5
    So h1/h2 = 3/4
    Volume1/ Volume2 = πr12h1/ πr22h2 = 12/25

You May Also Like

Current Affairs Quiz: April 30, 2024

CA Team

English: Word Usage Set 3

infoalfabanker

Quantitative Aptitude: Partnership Set 1

infoalfabanker
0